Equations

Author: r | 2025-04-24

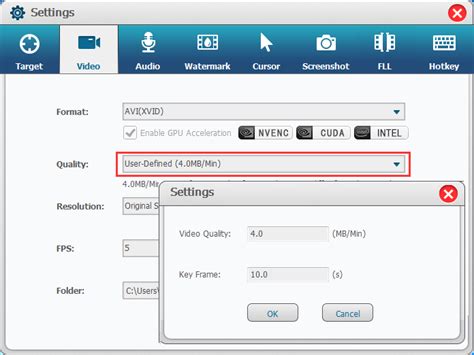
Polynomial equation. Linear equation; Quadratic equation; Cubic equation; Biquadratic equation; Quartic equation; Quintic equation; Sextic equation; Characteristic

Solution of equations and systems of equations

Jul 09, 2014 240 likes | 617 Views Solving Systems of Equations. Vocabulary. System of Equations: a set of equations with the same unknowns Consistent : a system of equations with at least one ordered pair that satisfies both equations. Download Presentation Solving Systems of Equations An Image/Link below is provided (as is) to download presentation Download Policy: Content on the Website is provided to you AS IS for your information and personal use and may not be sold / licensed / shared on other websites without getting consent from its author. Content is provided to you AS IS for your information and personal use only. Download presentation by click this link. While downloading, if for some reason you are not able to download a presentation, the publisher may have deleted the file from their server. During download, if you can't get a presentation, the file might be deleted by the publisher. Presentation Transcript Solving Systems of EquationsVocabulary • System of Equations: a set of equations with the same unknowns • Consistent: a system of equations with at least one ordered pair that satisfies both equations. • Dependent: a system of equations that has an infinite number of solutions; (lines are the same) • Inconsistent: a system of equations with no solution • Independent: a system of equations with exactly one solution • Solution to a system of equations: a point that makes both equations trueDifferent Methods for Solving Systems • Elimination Method: adding or subtracting the equations in the systems together so that one of the variables is eliminated; multiplication might be necessary first • Substitution Method: Solving one of a pair of equations for one of the variables and substituting that into the other equation • Graphing Method: Solving a system by graphing equations on the same coordinate plane and finding the point of intersection.Substitution Method STEPS: • Solve one of the equations for one of the variables • Substitute, or replace, the resulting expression into the other equation for the solved variable • Solve the equation for the second variable • Substitute the found variable into either

SOLUTION OF EQUATIONS AND SYSTEMS OF EQUATIONS

83x + 2PurposeEstablishes a relationship between two expressions or values, showing they are equivalent.Represents a value or combination of values that can be evaluated or simplified.SolvingCan be solved by finding the values of variables that make both sides equal.Can be simplified by performing arithmetic or algebraic operations.UseUsed in mathematical problems, modeling relationships, and real-world applications to find unknown values.Often used to create equations or to simplify and evaluate values.Important Notes on Equations in Math:Equations are a fundamental part of mathematics and have numerous applications across various fields. Here are some key points to consider:Defining Equality: Equations assert that two expressions are equal, creating a balanced relationship between variables, constants, and terms.Types: Equations come in various types, including linear, quadratic, cubic, polynomial, exponential, logarithmic, rational, trigonometric, and differential equations. Each type has unique properties and applications.Graphical Representation: Many equations can be visualized graphically, such as linear equations forming straight lines, quadratic equations creating parabolas, and cubic equations producing curves with multiple turning points.Solving Methods: Different equations require different solving techniques, such as algebraic manipulation, factoring, completing the square, the quadratic formula, Cardano’s method, or logarithmic transformation.Applications: Equations are used in various fields, including physics, engineering, economics, and computer science. They model relationships, such as distance-time-speed in physics, profit functions in economics, and algorithms in computer science.Systems of Equations: Multiple equations can form systems, which can be solved simultaneously to find common solutions. This is particularly useful in modeling complex relationships between variables.Real and Complex Solutions: Depending on the type of equation,

Equator Map/Countries on the Equator

Solve equations and inequalities Simplify expressions Factor polynomials Graph equations and inequalities Advanced solvers All solvers Tutorials Differentiate Basic Advanced Integrate Basic Advanced Partial Fractions Basic Advanced Matrices Arithmetics Inverse Determinant Simplify Basic Advanced Solve Basic Advanced Factor Basic Advanced Expand Basic Advanced Graph Basic Advanced Arithmetics Percentages Scientific Notation Expressions Simplify Expand Factor Equations Quadratics Solve Graph Inequalities Solve Graph Fractions Reduce Add Graph Equations Inequalities Welcome to Quickmath Solvers! Welcome to QuickMath main tutorials page.This is work in progress, so please keep checking! Linear equations Linear equations are the easiest ones to solve. Don't try any other equations before you master these with our tutorials and interactive linear equation solvers. Graphing functions Graphing functions is very similar graphing equations. Here you will learn proper functional notation as well as graphing functions that are more complex that the ones found in Graphing equations tutorials. Absolute value Are absolute values absolutely incomprehensible? Take a quick look at our step-by-step tutorials with interactive absolute value problem solvers. Roots and radicals Math roots worse than root canals? Not with our tutorials and interactive solvers. You will learn how to add, subtract multiply and divide roots as well as rationalize denominators. Exponents and polynomials Are you unsure about exponent rules, polynomial operations and similar topics? These tutorials will teach you the basic rules and operations, and our polynomial solvers will generate infintely many step-by-step solutions to a variety of problems Quadratic equations Quadratic equations are a little bit trickier than linear ones. If you are unsure about linear equations go over linear equations tutorials first. If you think you can handle them, use our quadratic equations tutorials and interactive solvers to learn about quadratic formula and other ways of solving second order equations. Complex numbers Are complex numbers too complex to learn? No, they are not if you use our complex number solvers and interactive tutorials. You will learn about why we need them, what an imaginary unit is, and a lot of other interesting things. Factoring polynomials Have problems with factoring? Which rule to apply when? Take a look at these handy interactive tutorials on different factoring methods. Fractions and rational expressions Are fractions giving you a headache? Learn how to reduce fractions and simplify a variety of rational expressions using our tutorials that include interactive fraction solvers. Arithmetic with integers and real numbers Need a bit of a brush up on. Polynomial equation. Linear equation; Quadratic equation; Cubic equation; Biquadratic equation; Quartic equation; Quintic equation; Sextic equation; Characteristic Screenshots Quick snapshots of UMS screens Equation Equation Equation Equation Equation-Hard Equation-Hard Equation-Hard Equation-Hard Function Graph Inequality Inequality

Word Equations to Chemical Equations

Arithmetics? Review the basics of arithmetics calculations and learn about representation of integers and real numbers using our interactive solvers. Graphing equations Graphing equations with our interactive graphers is easy! Not only do they let you graph equations, but with our tutorials you also learn about characteristic shapes and points of of common equations. Exponential function Is exponential function exponentially harder to learn than other functions? Not so with our interactive solvers, graphers and tutorials! Graphing inequalities If you already know how to graph equations, inequalities are easy-peasy. Lots of shading involved! Use our interactive graphers and tutorials to learn this important topic Linear equations in two variables This set of tutorials will explain how to solve and graph a system of two linear equations in a variety of ways Radical equations There is nothing very radical about radical equations! That is, if you know what a radical is. If not, go through our Roots and Radicals tutorial first. Then come back and use our interactive radical equations solvers and tutorials to learn how to solve this type of equations. Simplification of expressions This set of tutorials explains how to simplify a variety of expressions, such as fractions and radicals. Linear inequalities Good news: Solving linear inequalities inequalities is very similar to solving linear equations. Bad news: there is this pesky little inequality sign () that can change directions based on what you do with your inequality. Read our tutorials and use the absolute value solver to learn more.

Equation Wizard - algebraic equation solver

Ensure they satisfy the original equation.Types of EquationsEquations can be classified into various types based on their structure, degree, and the nature of their variables. Here are some key types of equations:Linear Equations: These are equations of the form ax + b = 0 where a and b are constants, and x is a variable. They graph as straight lines and have a degree of one.Quadratic Equations: These equations are of the form ax² + bx + c = 0 where a, b, and c are constants, and x is a variable. They graph as parabolas and have a degree of two.Polynomial Equations: These have the general form aⁿxⁿ + aⁿ⁻¹xⁿ⁻¹ + ... + a¹x + a⁰ = 0 where n is the degree of the polynomial, and each term includes a constant coefficient a and a power of x.Exponential Equations: In these equations, the variable appears as an exponent, such as in aˣ bˣ = c. Solving these often involves logarithmic functions.Logarithmic Equations: These involve logarithmic functions, such as log_b(x) = y, and can be rewritten into exponential form for easier manipulation.Rational Equations: These include rational expressions, such as fractions with polynomials in the numerator and denominator. An example is (x+1)/(x-1) = 3.Trigonometric Equations: These involve trigonometric functions like sine, cosine, or tangent, such as sin(x) = 0.5. Solutions often require knowledge of inverse trigonometric functions or identities.Differential Equations: These involve derivatives, such as dy/dx = 3x. They describe the relationship between a function and its rate of change,

Equation solver calculator - Rational-equations

Learning ObjectivesBy the end of this lesson, students will be able to identify and write balanced chemical equations, differentiate between different types of reactions, and interpret molecular models and reaction mechanisms to predict the outcomes of chemical processes. Students will also learn to write word equations, convert them into skeleton and balanced chemical equations, and understand the significance of ionic and net ionic equations. Additionally, students will gain the skills to classify reactions into synthesis, decomposition, single replacement, double replacement, and combustion reactions, and use molecular models to visualize chemical changes at the molecular level.Introduction Understanding how to represent chemical reactions is fundamental in AP Chemistry. These representations, including word equations, skeleton equations, balanced equations, ionic equations, and net ionic equations, provide a structured way to describe how reactants transform into products. Additionally, recognizing different reaction types and utilizing molecular models and reaction mechanisms helps in visualizing and predicting the outcomes of chemical processes.Types of Chemical Equations1. Word EquationsWord equations describe the reactants and products of a chemical reaction using their names. They are the simplest form of chemical equations and provide a straightforward description of the substances involved. Example: In this reaction, methane and oxygen react to form carbon dioxide and water.2. Skeleton EquationsSkeleton equations use chemical formulas to represent the reactants and products but do not indicate the relative amounts of each. This form is an intermediate step before balancing the equation. Example: Here, CH₄​ represents methane, O₂​ represents oxygen, CO₂​ represents carbon dioxide, and H₂O represents water.3. Balanced Chemical EquationsBalanced chemical equations ensure that the number of atoms for each element is the same on both sides of the equation, reflecting the Law of Conservation of Mass. This step is essential for accurately representing the reaction. Example: In this balanced equation, there are 4 hydrogen atoms and 2 oxygen atoms on both sides, ensuring mass conservation.4. Ionic EquationsIonic equations show the species that are actually present in the reaction mixture, including dissociated ions. This form is particularly useful for reactions occurring in aqueous solutions where ionic compounds dissociate into their constituent ions. Example: This equation shows sodium chloride and silver nitrate dissociating into their ions in aqueous solution, forming solid silver chloride and aqueous sodium nitrate.5. Net Ionic EquationsNet ionic equations show only the species that undergo a change during the reaction, omitting spectator ions that do not participate in the actual chemical change. This provides a. Polynomial equation. Linear equation; Quadratic equation; Cubic equation; Biquadratic equation; Quartic equation; Quintic equation; Sextic equation; Characteristic Screenshots Quick snapshots of UMS screens Equation Equation Equation Equation Equation-Hard Equation-Hard Equation-Hard Equation-Hard Function Graph Inequality Inequality